Composed Chess Studies: The Hidden Path To Improving

Written by IM Cyrus Lakdawala

Chess compositions (also referred to as studies or problems) are not positions from real games, but instead are composed or created chess positions or puzzles. There is a large network of players who compose these studies, who are referred to as chess composers or problemists.

Some chess studies could occur in real games, while others are very unlikely (or even impossible) to ever appear on the board in a real game. Here is an example of the latter, which is Black to move and mate in two:

As you can see, the likelihood of this position occurring in a real game is zero. However, it shows a very distinct and simple pattern that absolutely can help in your real games! The solution is 1...Nxe3 2.Rg4 (the only legal move for White!) Nxc2#.

Black to move-Mate in two

Most players dismiss chess compositions solely because some of the positions could never happen in their own games—but they are wrong to do so! If you can solve difficult and crazy-looking compositions, then you can solve any "normal" position that you'd ever be faced with. 

I retired from tournament chess in 2019 and just for fun, attempted to solve composed endgame studies and composed mating problems on a daily basis. Then in a few months, I noticed that my online blitz and bullet ratings began to climb—I found this odd since I was 59-years-old, at that time, and very few players rise in their chess dotage. So I began an experiment and invested half my lessons with students on endgame studies and composed mating problems, guiding them through problems and studies which if attempted alone, were way past their tolerable complexity levels.

Within six months, I witnessed shocking jumps in some students. One example is a student named Jonathan who plays at the San Diego Chess Club, then age 55, and who for the last 20 years cycled between the ratings of 1290 and 1360. Jonathan worked a daily regimen of composed mates in 2 and also worked on endgame studies.

The endgame studies were way too difficult for him. So the system was for him to try to solve for a few minutes, then if he didn’t have a clue on how to proceed, simply look up the answer. Within six months, his rating climbed to 1576—over 200 points higher than his former peak rating, and I bet he would have broken the 1600 barrier if the pandemic hadn’t arrived and over-the-board chess didn’t come to a halt.

In 2019 I began the Facebook group Chess Endgame Studies and Compositions with my friend Australian GM Max Illingworth, a former Chess.com coach of the month. In less than two years, we picked up more than 26,000 members and are growing at a rate of around 1,000 to 1,500 members per month. It seems like a lot of people understand how fun and valuable chess problems are.

Facebook users are not the only ones who enjoy these studies, though. The training benefits of endgame studies have been known for half a century in the old Soviet Union. Top trainers like IM Mark Dvoretsky and now India’s most decorated trainer GM Ramachandran Ramesh use endgame studies to train students. Endgame studies contain every aspect of chess. In order to solve them:

1. We strain to find a plan which in most cases doesn’t even appear to exist. We envision a lengthy and complicated chain of events whose completion is for the moment an abstract concept, without flesh, blood, or bones.

2. Then we need to calculate with engine-like precision to implement the plan. One slip in the calculation and your solution comes tumbling down.

3. Finally, our assessment must be correct in the aftermath. Your calculation can be perfect and you reach your desired position. Except that it isn’t the solution! So we stop guessing (as we so often do in our games) with thoughts like “This move seems to work.” That lazy, intuitive thought sinks us when attempting to solve composed works. If our guesswork fails, then we fail along with it.

In our tournament games, many of us sometimes attempt to positive-think our way to the solution. There is such a thing as toxic positivity, where we attempt to will our way to the solution, rather than work it out in precise, mathematical detail. Working on endgame studies breaks this common habit.

I say again: you don’t need to successfully solve them. All which is required is that you try for a few minutes and if nothing pops up, simply look up the answer. After a while, your internal database fills with startlingly original geometries which you just will never see with conventional chess puzzles (although we should drill in these on a daily basis as well).

The alchemic transformation of iron to gold was one of the great medieval dreams. Try an experiment and incorporate composed works in your daily training for six months and see if there is an alchemic change in your pattern recognition.

What is so special about endgame studies and composed mating problems? Let’s take a look and you judge for yourself:

R.Reti-White wins 

Bohemia, 1923

You are playing this position as White against an opponent who outrates you by 300 points. You were easily winning earlier. Then depressingly, your opponent outplayed you to reach this drawn position. But it’s not so bad since a draw against an opponent 300 points higher is still a great achievement. It is a draw, right?

After all, there is no way to stop Black's promotion without giving up our rook, correct? There is no way you can give up only your bishop for the pawn and obviously, there is no way to mate Black's king. If you thought this, then you assessed the position incorrectly! White is winning, but only if your mind is attuned to the position’s hidden mating geometry. "Mating geometry, with this little material on the board?" you ask. 

Exercise (combination alert): Look around. There is a way for White to either mate Black or only give up the bishop for Black's final pawn and win with king and rook against the lone king:

When it comes to chess teachers and writers, we all believe we know what is best for students and readers. So here is my advice: Attempt to solve composed endgame studies and stipulated mating problems, like this one. You don’t need to be correct. Just go through the process of trying (usually failing) and then looking up the answer.

After a while, we accumulate a warehouse of new geometries, which translates to seeing more combinations, deeper and with greater clarity in our over-the-board or online games.

Let's take a look at another position:

W.Lewis-White draws

Chess Problems #97, 1827

How can this possibly be drawn?

1. White is down two pawns.

2. Black enjoys an advanced king position over White's king.

3. Black owns bishop against knight, generally considered an advantage in most endings. 

Does White have anything at all? Well, there is one prayer: if we can somehow give away our knight and c-pawn for Black's d and b-pawns, then we draw, because rook pawn and bishop against the lone king is drawn with the following two factors present:

1. If the rook pawn's promotion square is the opposite color of the bishop. Notice that a1, the rook pawn's queening square is a dark square, while Black's remaining bishop sits on a light square.

2. The defending king must be able to reach the corner, in this case a1. Can we accomplish this?

Pretty neat, huh? Try this next one on for size:

O.Duras-White wins

Sachové Listy, 1901

If any of you read Edward Lasker's classic Chess Secrets I Learned from the Masters, then you are familiar with Oldrich Duras, who was a top player in his day. And like Richard Reti, was also a superb endgame study composer. The knowledge acquired in one study may help us solve another. We learned about rook pawns and bishop of the wrong color in the last study. Keep this in mind when attempting to solve this one.

We have a big problem, despite our extra bishop, since our useless pawns are stuck on the rook file, and guess what? Our promotion square on a8 is the wrong color as our remaining bishop, which we now know is a draw. Look deeply and we may find White's mating pattern:

Pure Awesome! Let's move on to another:

A.Troitzky-White wins

Zadachy i Etyudy, 1930

Let's try and understand what we are up against:

1. Black threatens to draw immediately with ...Bxf2 and if we move our knight, our h-pawn falls.

2. I envision a mate with the white king on f5, the black bishop on h4 and we can play either with Be2 mate or Nf4 mate. 

3. The problem with this plan is: how do we induce Black's bishop to remain on h4? Another problem is that Black is by no means obliged to take on h4 with the bishop. Black can play ...Kxh4.

4. The only way I saw to force ...Bxh4 was to attack it with 1 Nd3, which as it turns out is the correct first move of the study:

If this were a movie we would hear the strains of sweet, uplifting music which would show how the hero triumphed, against all odds.

Are you tired yet, or do you want more? OK... here is a mate in two:

G.Legentil-Mate in two

Journal de Rouen, 1926

When I post mate in two problems in the Facebook group, where White is up a massive amount of material, I get complaints like this one: "This problem is stupid! Why watch a sporting event when you know in advance the side who will win? After all, White can make any old move and still win. Why should I sweat to fulfill a phantom mate in two deadline when I can easily mate in five, or win the opponent’s queen?"

Such complainers completely miss the point behind stipulated mating problems. The idea isn't: White to play and win. Of course, White is winning by an overwhelming margin. The challenge is to deliver mate in two moves and no more. If you spot 15 mates in three and miss the single mate in two, you failed the problem.

What is the point then of solving such stipulated problems? Imagine this: in your tournament game you began an attack on your opponent, and sacrificed a piece and three pawns. Embedded within the position is a hidden mate. Find it and you win; miss it, you lose. This is exactly where stipulated mating problems come in. You become accustomed to threading the needle, where you find the single path, where all others fail. 

You become accustomed to threading the needle, where you find the single path, where all others fail."
— IM Cyrus Lakdawala

In each of these problems, Black's only goal is survival past the stipulated number of moves:

Did you like that one? Well, here is another mate in two:

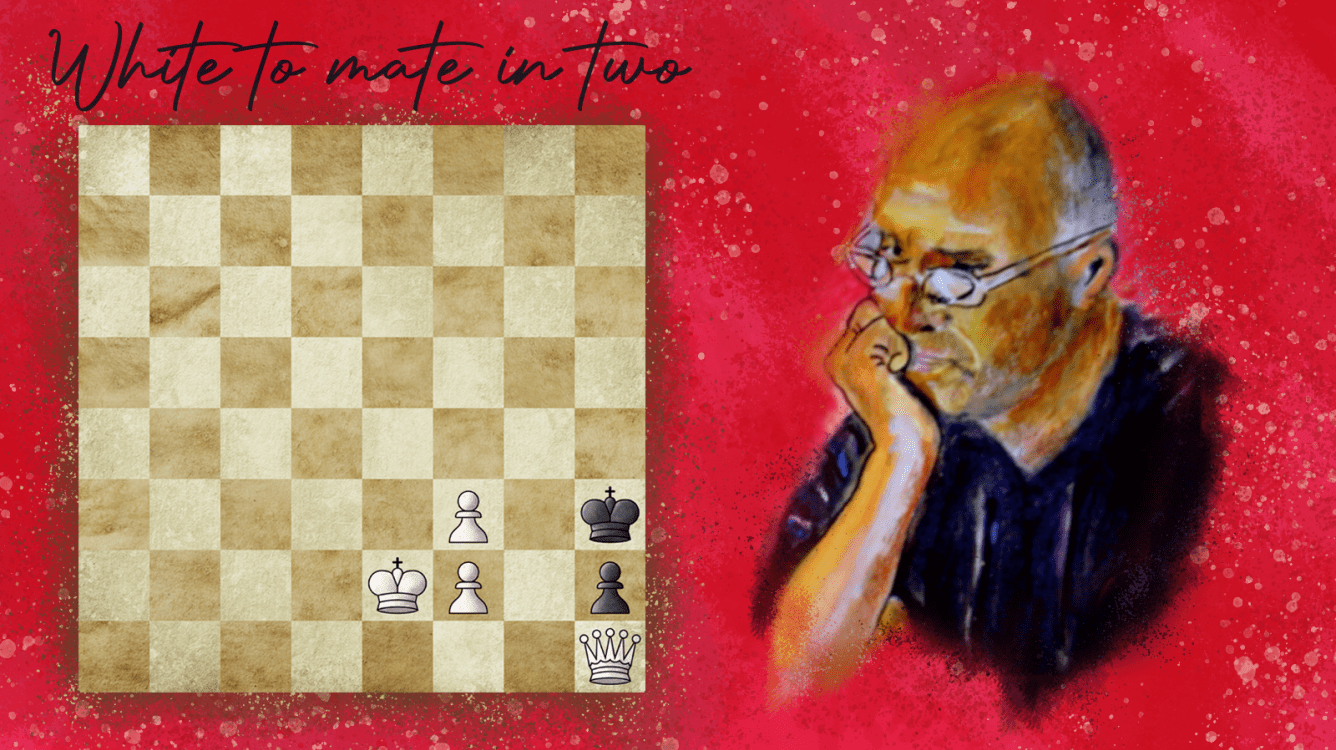
S.Dowd-Mate in two

Chess Endgame Studies and Compositions, 2021

Not every study or mating problem is filled with thrills and melodrama. This one is straightforward. Professor Steven Dowd is a friend and one of the administrators on my Chess Endgame Studies Facebook group. He also happens to be one of America's premier mating problem composers. Dowd was kind enough to compose an easy yet elegant study for us.

It's obvious that our first move must be a queen move. But to which square?

That was a lovely one—do you like mate in two problems? OK, one more then:

F.Giegold-Mate in two

Schach-Eco, 1963

Look for White's least likely move and there you have your answer. In our tournament games, we are accustomed to winning via a gradual, linear process of accumulation. This is not how composed mating problems work! Do you see White's impossible-looking next move, which forces mate in two?

Alright... Let's try some mate in three studies. Here is the first:

W.Atkinson-Mate in three

Canadian Chess Problems, 1890

Does this position look familiar? It should because it is the one Benny and the other gradmasters used to test Beth Harmon in the Netflix Queen's Gambit series. It's a White to mate in three problem, which Beth solved in seconds. How did her intuition come up with the answer so quickly? Her intuitive mind instantly processed the following data:

1. White is up two pieces.

2. Black's king is seriously restricted, currently with access to only f6 and g7.

3. White's b7-knight is not participating in the attack. In composed mating problems and endgame studies, there is not even a molecule of redundancy on the chessboard. Every piece has its specific function.

4. So the mate clearly involves the b7–knight. But how do we activate it to relevance? The d8–square covers e6 and f7, which are already covered, so it's not needed there. If we move to c5, the knight check on d7 doesn't get the job done either since Black's king runs to f7.

5. Then Beth notices that if the b7–knight were magically moved to e8, it would deliver mate. The only route to e8 is to move the king to d7, clearing d6 for the knight and where the king continues to cover the e7–square. And there we have our answer!

This is cinematic stuff! Here is our last study for this article—another mate in three:

F.Giegold-Mate in three

1952

This is a stunningly elegant construct. Black has only one legal move available on each turn. So we deduce that Black's next two moves will be 1...f5–f4 and 2...Kf5. What you need to do is visualize a formation where the white bishop and rook force mate in that position.

We all dream of perpetual progress and unlimited rating abundance. If you seek to move in that direction, then consider endgame virtuosity via composed studies. You may not agree with this assessment, but in my opinion, Jose Raul Capablanca, GM Bobby Fischer, and GM Magnus Carlsen all became world champions in large part to their domination over their rivals in the endgame phase.

They saw subtle patterns, hidden from their colleagues. Endgames teach us that there is tactical life in even the most barren of wastelands. Add composed mates in two, and your calculation ability and tactical insight may rise. I touched upon this idea in my book titled First Steps: Fundamental Endings and expanded upon the same idea in my recent publication, Tactical Training in the Endgame.

Do you enjoy composed problems? What was your favorite study in this article? Let us know in the comments below!