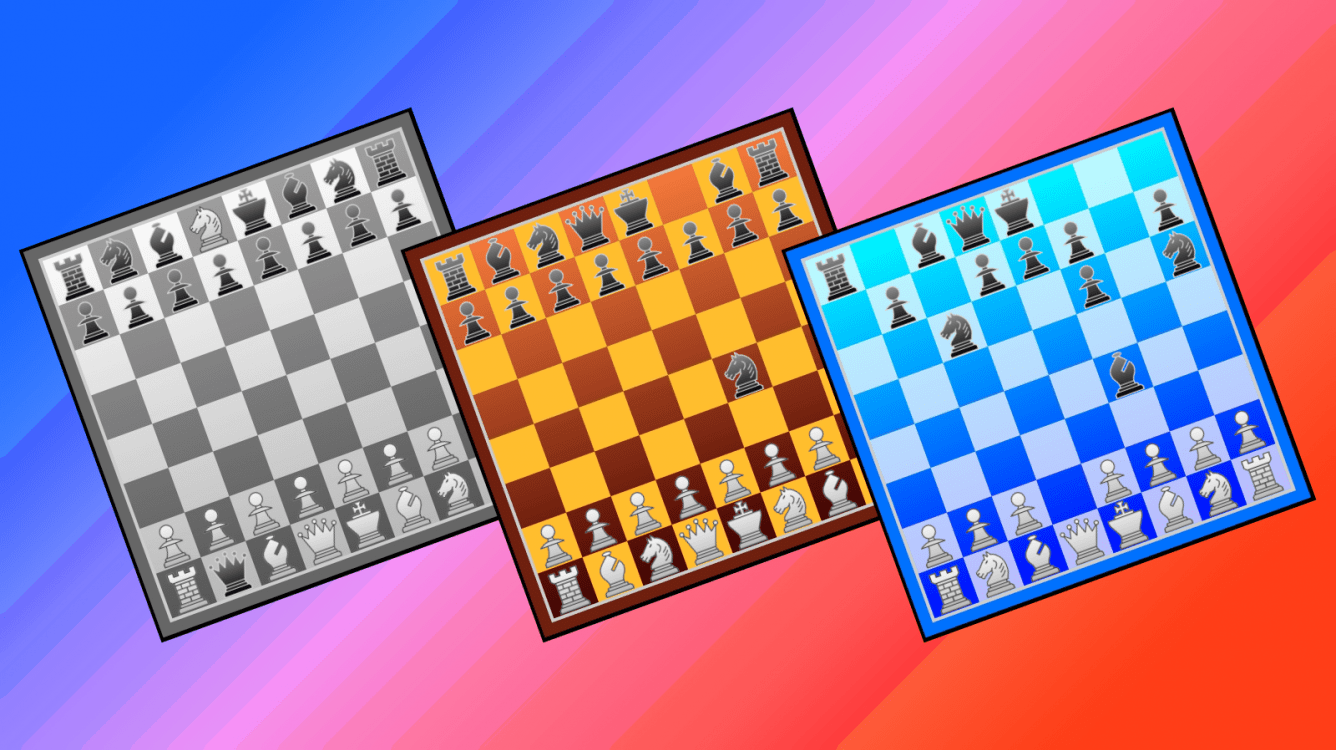
Christmas problem-solving competition
The festive season is upon us, so let’s celebrate with a fun problem-solving contest with prizes! It’s something of a Christmas tradition for chess problemists to send one another greeting cards diagrammed with their own compositions – often of the unconventional variety. In keeping with that custom, here are three problems I’ve devised that may involve some thinking outside the box. Solve at least one of these positions for the chance to win one of three prizes: your choice of a Chess.com Diamond membership or a Redbubble.com gift certificate. More details are found at the end of this blog.
1. Miraculous setup
We start with a quirky setup where a white piece and a black one are swapped in the initial array. Yes, it’s an illegal position but hey, it’s Christmas and anything is possible! Other than that, this solves as a standard helpmate problem. The idea of helpmates is fairly straightforward: Black plays first and the two sides cooperate to enable White to mate as quickly as possible – in 2 moves in this case. The Fool’s mate can be viewed as a potential answer here, but 1.f6 e4 2.g5 Qh5 doesn’t work, obviously because d8 isn’t blocked by the queen. The duplex condition means that you also need to find a second solution in which the two players reverse roles, i.e. White plays first and helps Black to give mate in 2 moves. For another example of a helpmate-in-2 (also a duplex), see the second problem in A tribute to Pal Benko.
2. Shuffling pieces
In the popular Chess960 variant (aka Fischer random chess), the two players start the game with the main pieces randomly placed (and mirrored) on their first ranks. Suppose that the above position arose in such a game, where each player’s bishop-and-knight pairs were originally interchanged. Now apparently there is a mate-in-1 for Black, but is it actually a legal move? In other words, is it really possible that it’s Black to play in the diagram? To solve this one, put on your detective’s hat and work out what could have occurred in the game, regardless of how unlikely any moves may have been. Provide a short explanation or proof for your answer, whether it’s “yes” or “no”.
3. Two impostors
In a proof game problem, your task is to reconstruct the legal game that led to the diagram position, starting from the normal array. The shortest possible game is required and you are given its number of moves – here 10 by each side. That the correct moves would not be sensible in a practical game is considered irrelevant. Note that the solution of a proof game is a unique sequence with no possible alternative moves, and that can be a hint in itself. In the above position, Black has doubled f-pawns and White is missing only the d-pawn, but the latter apparently couldn’t have made two captures to reach f6. So what was captured on f6 and what happened to this d-pawn? The title of this problem, “Two impostors,” refers to pieces with surprising identities in the diagram.
To enter this competition, send your solutions to me via Messages on this site. A correct solution to each problem earns one point, and your total number of points determines how many times you go in the draw for one of the three prizes. So cracking all three problems will give you more chances, but you can still win by solving any one of them. Each of the three winners can choose either a Chess.com Diamond membership (one month) or a Redbubble.com gift certificate valued at 30 USD. All sorts of merchandise are available from the latter site, including some with chess designs made by yours truly.
To be fair to all entrants, I won’t comment on the correctness of your answers while the competition is still open. Of course, please don’t post any spoilers or hints in the comments below or anywhere else. The closing date is Jan. 5, 2020, and good luck!
Christmas problem-solving competition – solutions and results


